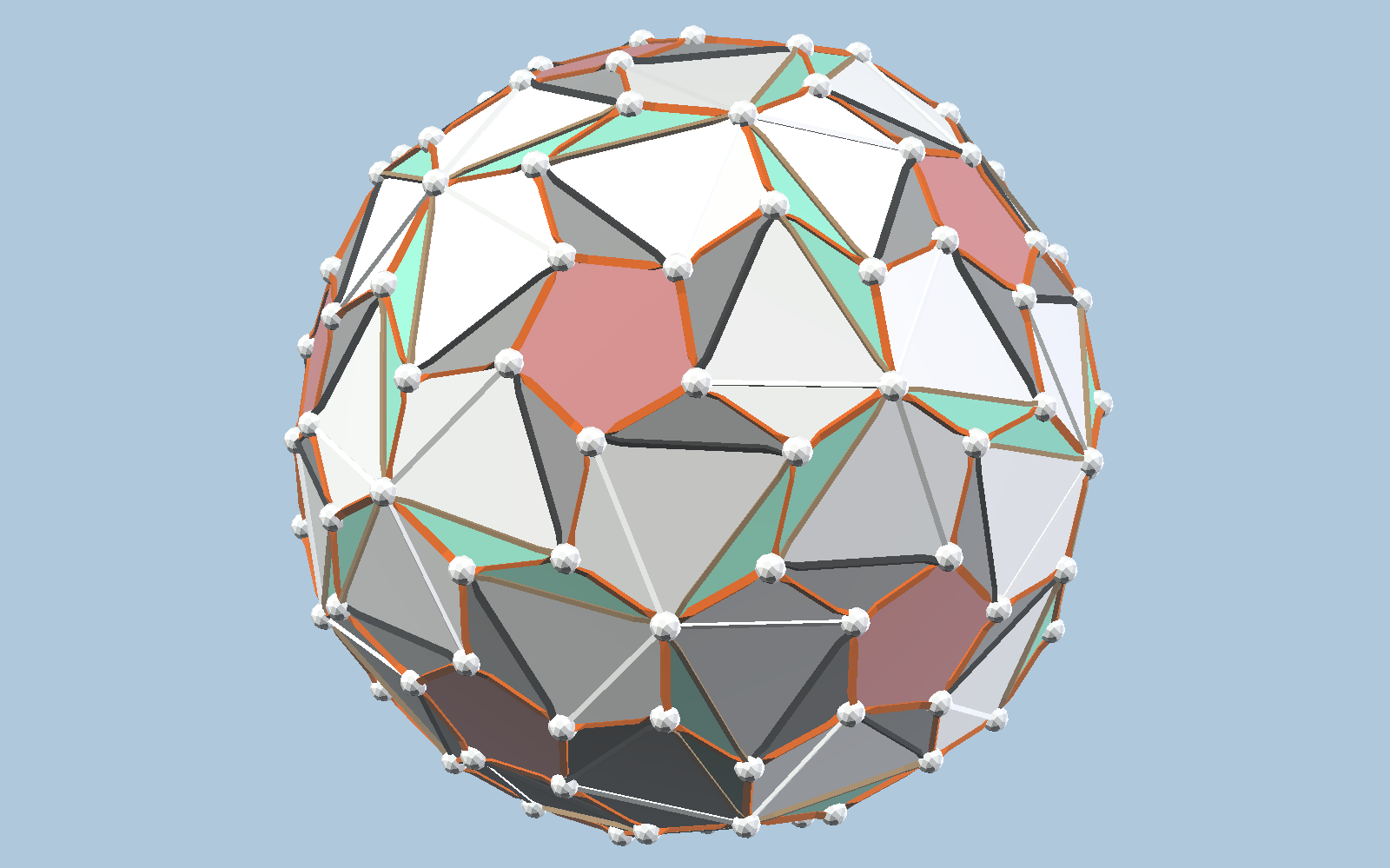
This is near-miss for a Goldberg polyhedron, as described by Schein and Gayed in 2014.
I found this design while experimenting with my new orange struts in vZome in 2006. It is very appealing, as an all-orange construction, possibly modeling a kind of Buckminsterfullerene with 140 carbon atoms.
In 2014 Stan Schein and James Gayed published their groundbreaking paper on Goldberg polyhedra. At first I thought my orange polyhedron fit the bill, and I even sent them the design, congratulating them on their discoveries. Stan and James had been using vZome since 2012, and we had corresponded a bit, though I don’t think vZome was specifically useful for their modeling needs.
In their notion of Goldberg polyhedra, there are several important criteria. The first is equilaterality, having all edges the same length. This criterion is clearly met by my orange design, being constructed from a single size of orange strut. The second important criterion, however, is not met, that of convexity. An equivalent (I think) way to state this is that the polygon faces must be planar. A simple application of vZome’s “convex hull 3d” tool confirms that my design does not have planar faces: